Log-Normal Distribution

“Well asset prices follow a lognormal distribution, which is skewed to the right because asset prices are non-negative. Hence they are positively skewed! Most good stocks are positively skewed. “

Let’s revise a-bit with Positive Skewness –

• Conversely, data that has a positive skewness is said to be skewed to the right.
• In case of positive skewness (green curve here), the data piles up on the peak on the left side and the tail points right.
• Positively skewed returns mean frequent small losses and few large gains.

In probability theory, a log-normal (or lognormal) distribution is a continuous probability distribution of a random variable whose logarithm is normally distributed. Thus, if the random variable X is log-normally distributed, then Y = Log (X) has a normal distribution.

A random variable which is log-normally distributed takes only positive real values as Log (X) cannot return a negative value. The distribution is occasionally referred to as the Galton distribution.

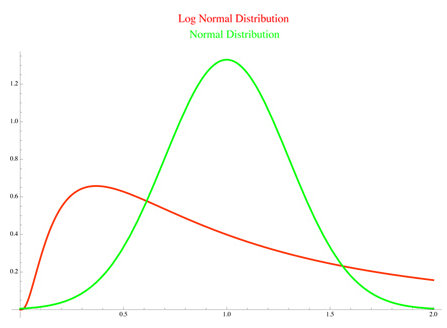
Stock markets returns are defined by Log (T/P) and hence follow a log-normal distribution.

It’s more realistic than normal distribution hence but we assume a normal distribution in our models to make our life easier.